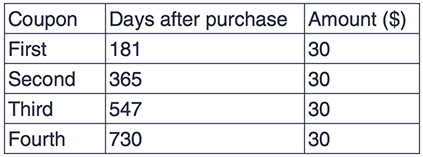
An investor bought a newly issued bond with a maturity of 8 years 150 days ago. The semi-annual coupon rate of the bond is 6% and the face value is $1,000. Currently the price of the bond with accrued interest is $1,026.5. The investor plans to sell the bond after 365 days from today. The schedule of coupon payments for the first two years from the date of purchase is as follows:
Assume a 4% risk free interest rate and that the investor is taking a short position in the forward contract at no-arbitrage price. After 90 days of entering into the contract, the price of the bond including accrued interest is $1,024.75. The value of the forward contract shall be closest to
A ) $ 59.05 loss
B ) $ 19.05 gain
C ) $ 19.05 loss
Explanation of Answer
S0 = $ 1,026.5 T = 1 r = 4% PV of coupons = 30/(1.04^(31/365)) + 30 / (1.04^(215/365)) = 59.22 No-arbitrage price of forward contract = (1026.5 – 59.22)*(1.04^(365/365)) = 1005.97 Bond price after 90 days = 1024.75 Forward contract price = 1005.97 t = 90/365 and T = 365/365 = 1 We are at 240th day of bonds life. One more coupon of $30 shall be paid before the expiry of the forward contract. PV of coupon = 30/(1.04(307/365)) = 29.03 Value of forward contract after 90 days = 1024.75 – 29.03 – 1005.97/(1.04(275/365)) = 19.05 $19.05 shall be the loss to the investor as he is the seller.
My question is that why will the coupon on day 547 be discounted to present value for calculating the value of forward contract at 90 days into the contract? PV of coupon = 30/(1.04(307/365)) = 29.03 should not be included in the calculation in my opinion as 150+365=515 and hence the coupon payment at 547 day will not be collected. Please let me know how that is included in the explanation?